Sound is something that all of us have heard about. What we perceive as sound is really variations in the air pressure on the eardrum. These variations propagate deeper into the ear as vibrations and then into the brain as electrical impulses. Pressure is therefore central to acousticians and everyone else working with sound.
In this post, I want to tell you about where air pressure comes from. This also tells us something about how the nature of air pressure limits how good it is possible for ears and microphones to become.
Gas molecules
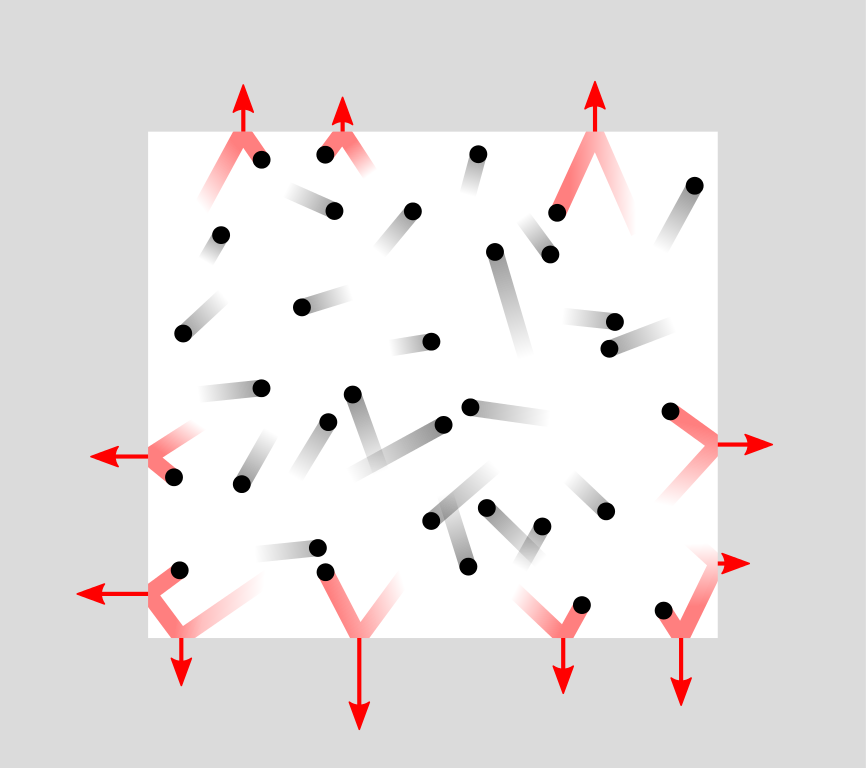
It is tempting to think of matter, such as a droplet of water, as a continuous blob of material. This is called a continuum. But this is not how reality works. We have known for more than a century that all materials are made up of molecules. (In turn, these consist of atoms that consist of subatomic particles, but let’s not get too distracted.) In a gas such as air, these molecules zoom around and collide with each other. The adjacent animation gives you an idea of this.
When an air molecule bounces off a surface, this changes the velocity of the molecule. This means that a small amount of momentum (i.e., mass times velocity) has been transferred from the surface to the molecule. According to Newton’s third law of motion, the molecule has simultaneously transferred a small momentum to the surface. It’s like throwing a marble at a wall: The marble bounces off, bumping the wall as it does.
Pressure is force per area
Every second, a wall gets an incredible amount of such tiny bumps from air molecules bouncing off it. Together, these bumps excert a fairly steady force on the surface. A larger surface gets bumped by more air molecules and the total force on it is therefore larger, but the pressure, which is force per area, is the same.
To measure air pressure directly, we need a surface on which the air can push. In a barometer, this is typically a plate or a liquid surface. In microphones and on the eardrum, air molecules bounce off a membrane that can quickly react to pressure changes.
The pressure of a gas increases with its density. (The more molecules, the more bumps a surface gets.) It also increases with the speed of the molecules. (The faster they are, the harder they bounce.) One equation that shows this directly is the ideal gas law,
\(p = \rho R T ,\)
where \(p\) is pressure, \(\rho\) is density, \(T\) is temperature, and \(R\) is the specific gas constant. This equation shows us that the pressure increases proportionally to density and temperature, and temperature is closely related to the average speed of molecules.
Variations in pressure
OK, so air consists of molecules. Very, very many molecules. In a single cubic centimeter of air, there are tens of billions of billions of molecules. These zoom around in a fairly chaotic motion. (In fact, the words “gas” and “chaos” both come from the ancient Greek word χάος.) As the above animation indicates, this chaos means that there is a bit of randomness in how many molecules hit a given surface every second. Therefore, air pressure is not completely steady; it varies a tiny bit.
The law of large numbers says that the relative effect of such random variations decreases as the amount of events, such as molecules bouncing off a surface, increases. It’s like tossing a coin a thousand times. You will probably get a fairly even distribution of heads and tails. On the other hand, if you throw a coin, say, four times (or in the most extreme case, one time), the distribution of heads and tails will tend to be much less even.
Sensing pressure variations
Even though there are incredibly many molecular collisions against the eardrum every second, these variations can in principle be sensed as noise. As the Nobel prize winner Richard Feynman said in his Feynman Lectures on Physics,
If our ears were a few times more sensitive, we would hear a perpetual rushing noise. Evolution has not developed the ear to that point, because it would be useless if it were so much more sensitive—we would hear a perpetual racket. The reason is that the eardrum is in contact with the air, and air is a lot of molecules in perpetual motion and these bang against the eardrums. In banging against the eardrums they make an irregular tattoo—boom, boom, boom—which we do not hear because the atoms are so small, and the sensitivity of the ear is not quite enough to notice it.
So, how sensitive would the ear need to be for us to be able to hear these random pressure variations? Different sources disagree a bit, but it looks as such the noise level on the eardrum is around -24 dB. That is, about 24 dB weaker than the weakest sound that we can hear. Thus, the human ear is quite a way away from being limited by this effect, but the most sensitive cat ears may be close.
This effect also limits how sensitive microphones can be at a given size. The smaller the microphone membrane is, the fewer molecular collisions it experiences every second. According to the law of large numbers, fewer collisions increases the random variation of the pressure. Therefore, it is simply not physically possible to make a tiny microphone that is extremely sensitive, even assuming perfect noise-free electronics.
Sound
In this post, we considered where steady pressure comes from, and how the random motion of air molecules causes pressure variations that in principle can be sensed. Sound, on the other hand, is variations in the pressure due to waves of compression and rarefaction. If you want to learn a bit more about that and how we can describe sounds, I suggest having a look at my blog post on decibels.
This blog post is a translation of a Norwegian-language blog post that I wrote for the Acoustics Research Centre blog in 2015. All pictures taken from Wikimedia Commons.